The method of simplifying consists on transforming one expression into another one simpler, more useful to work, or easier to memorize. We simplify fractions, polynomials, powers and radicals... It's a great Mathematical tool, but it can become a dangerous method if you don't use it properly.
(This post participates on the 127th edition of the Carnival de Mathematics, hosted by the blog Mathematics and Coding.)
FIRST HALF
 Real Madrid president feels anxious. He’s not able to balance the books of the sales in the stores.
Real Madrid president feels anxious. He’s not able to balance the books of the sales in the stores.After the signing of Iker Casillas for Porto, Florentino wants to clear the stock of the goalkeeper t-shirts as soon as possible.
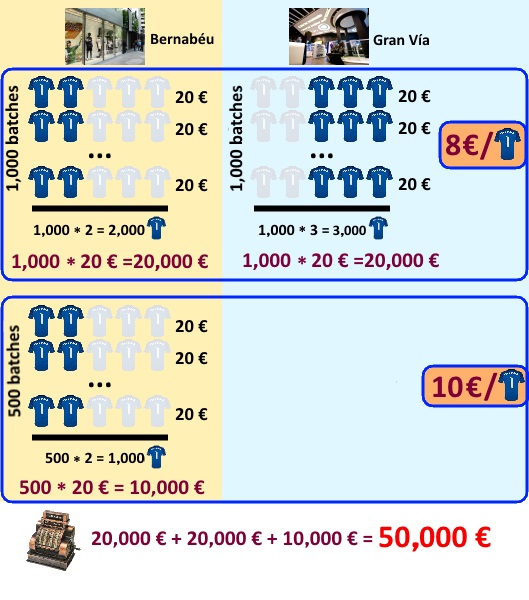
To achieve this, he has instructed the two official stores. In the store closer to the Santiago Bernabeu stadium, they will sell 2 t-shirts for 20 € in total, while in the other store, the deal will be 3 t-shirts for 20 €.
In the first week, they have sold a total of 6,000 t-shirts, 3,000 in each store. In total, they have made 50,000 €.
But there are still many shirts to sell, so in the second week they have decided to change the offer.
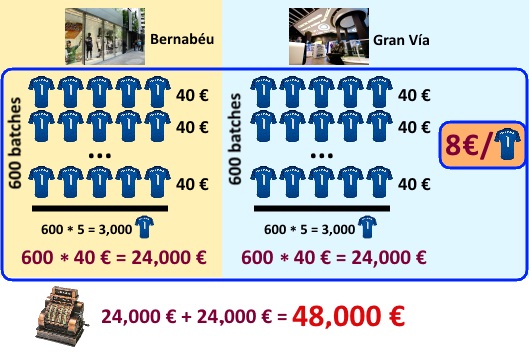
If the previous week they sold 2 units for 20 euros and another 3 units for 20 euros, now both stores will sell batches of 5 t-shirts for 40 euros, and will get the same income for every 5 t-shirts.
During the second week, they have managed to sell the same number of shirts (6,000), also 3,000 in both stores, but the takings have dropped to 48,000 euros.
The club doesn’t understand why they have got less money. They think that maybe an employee of one of the stores has stolen some notes.
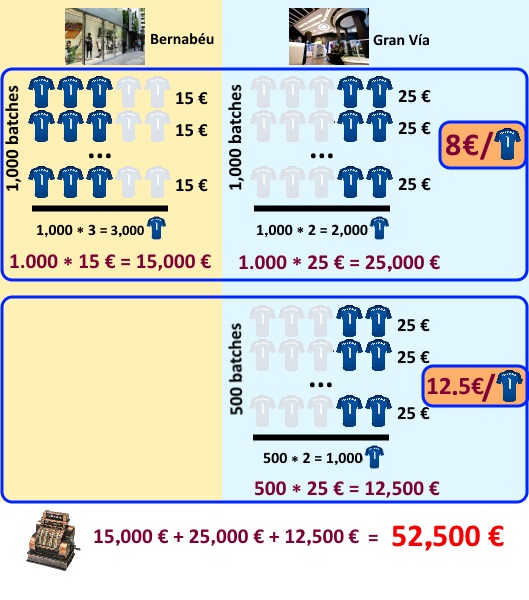
 The fact is that there are still 6,000 Iker t-shirts to sell. This time it's been decided a different deal. They’ll sell batches of 3 units for 15 euros at the shop of the stadium, and batches of 2 units for 25 euros at the store of Gran Vía. Thus, for every 5 shirts they will get again a total of 40 euros.
The fact is that there are still 6,000 Iker t-shirts to sell. This time it's been decided a different deal. They’ll sell batches of 3 units for 15 euros at the shop of the stadium, and batches of 2 units for 25 euros at the store of Gran Vía. Thus, for every 5 shirts they will get again a total of 40 euros.Finally, they sold the remaining 6,000 shirts, 3,000 in each store again. But this time they get 52,500 euros.
Now the board of directors doesn't understand anything. It’s possible that the employee who stole 2,000 euros last week has returned them to the cash desk, or maybe the money was miscounted last week. But then, what about the extra 500 euros of the third week?
Undoubtedly, this is a mystery that only Joe Vitruvius can solve, so Florentino calls him to come and to solve the enigma.
SECOND HALF
 - Hi, Florentino, how are you?
- Hi, Florentino, how are you?- Well, we’ve got a small problem. We sold 18,000 t-shirts over the past few weeks. We have asked 40 € for every 5 shirts, so we should have entered 144,000 euros. And yet, we got 6,500 euros more.
- That's fine. With that extra money you can sign a new goalkeeper, right? Or you can also buy a next-generation fax...
- The thing is that during the last three weeks we have sold the same number of t-shirts and yet, every week we have cashed up a different amount of money.
- I see. Were all units sold at the same price?
- No. We have two stores, and each one had a different offer.
- Well, it may explain everything.
- No, because for every 5 t-shirts, the money extra we get in one store equals the money we lose in the other.

- Then it's clear. You've sold more t-shirts in the store where you have a better deal. That’s the difference.
- Not either. It turns out that despite the offer, we sold the same number of shirts in both shops. So the differences weren't because we sold more shirts in the cheapest store.
I think that there is an employee who wants to turn us crazy with this, perhaps a staunch supporter of Iker who didn’t like him to leave Real Madrid, or something like this. I would like to find the saboteur.
- Well, I think that such a person doesn’t exist. It's just a small mathematical paradox, because of the simplification on the calculus.
- Oh yeah? What is it about?
- Let’s see. We’ll start with the central week, in which both stores sold batches of 5 t-shirts for 40 euros. If 6,000 shirts were sold in total, we can say that 1,200 batches of 5 units were sold, or what is the same, you sold t-shirts for 8 euros, right?
- Yes, I think it's pretty clear.
- Now let's see what happened on the first week.
 - Then we also sold batches of 5 t-shirts for 40 euros. In the store close to the stadium we sold 2 t-shirts for 20 euros, and in Gran Vía we sold the remaining 3 shirts for 20 euros.
- Then we also sold batches of 5 t-shirts for 40 euros. In the store close to the stadium we sold 2 t-shirts for 20 euros, and in Gran Vía we sold the remaining 3 shirts for 20 euros.- There's the trap. In this case we can’t talk about batches of 5 shirts, since it’s not entirely true. You are wrongly simplifying the distribution of your sales.
- Why?
- In each store you sold 3,000 shirts, right?
- Certainly.
- In this case we can only speak of 1,000 batches of 5 t-shirts (sold at an average of 8 euros each unit). And a surplus of 1,000 t-shirts, which were sold in the store close to the Bernabeu stadium at 2 shirts for 20 euros, ie 10 euros per t-shirt.
Therefore, part of the t-shirts were sold at a higher price. That’s why you got an additional money during the first week.
- And if you look at the last week, we can see that a total of 1,000 batches of 5 t-shirts were sold for a total of 40 euros (at an average of 8 euros a t-shirt). And there were still 1,000 t-shirts in the store of Gran Vía, which were sold at 2 units for 25 €, ie 12.5 euros per shirt.
And that's why this week you got even more money.
- Now I understand everything. So there is no saboteur...
- Nope. The error was that you thought that the t-shirts were sold in batches of 5 units all the time, when it happened only one week.
- And has this ever happened elsewhere?
- Yes. The writer Malba Tahan, in his book 'The man who counted', writes a case just like this with some pineapple vendors. And Martin Gardner, on his book 'Aha! Gotcha. Paradoxes to puzzle and delight', shows a similar case which takes places in a music store.
 -OK, now I stay calmer. We'll have to see what we can do with the extra money we have got.
-OK, now I stay calmer. We'll have to see what we can do with the extra money we have got.- Well, you can give the extra money to any philanthropic association. Or you can also buy some books of recreational mathematics, and give them to the children who come to the stadium, at the beginning of the next match. Surely you will increase the attendance to the stadium.
- We will think about it. Bye, Joe, and thank you very much for your help.
- Till next time, Florentino.

And don't forget to take a walk by the 127th Carnival of Mathematics. There you'll find lots of excellent math posts that you'll surely like too.