A player has been killed in the locker room at halftime of a match of the World Cup Brazil 2014. A series of mathematical clues leads you to solve the mystery, with the help of inspector Larry Flanagan and official Joe Vitruvius, that will introduce the fascinating world of Combinatorics.
Do you dare to solve the case?


(This post participes on the 112th edition of the Carnival de Mathematics, hosted by the blog Theorem of the Day.)
The mathematical murderer
Nobody could believe it. They were waiting for him to begin the second half of the match, but he didn’t go out to the pitch. Finally, the physical trainer went down to the changing room to see what was happening, and found him there, lying on the floor, with a dagger in his chest.
It was Drakulic, the center forward and captain of the national team of Transylvania, that had brilliantly qualified for the first time for a final tournament of a World Cup, that this year Brasil holds.
None of the players remembered who had been the last one to leave the locker room, because they were very upset by the talk of their coach, Pep Tourinho, as they were losing at halftime by 2 goals to 0 with the national team of Western Micronesia.
The organizers of the Championship wanted the case to be solved quickly and discreetly, so they requested the Police for their best officials, so as to solve the case as soon as possible.
In few minutes, inspector Larry Flanagan, and agent Joe Vitruvius, from the Mathematics Department of the Police, arrived to the stadium.
Why were they chosen? Well, Larry Flanagan (F) was the most brilliant inspector of the police. But he wasn’t very good at Mathematics.
The collaboration of an official of the Mathematics Department seemed essential because, stuck on the dagger, there were three scraps of the today’s newspaper, all of them related to the match they were going to play, and with some mathematical notations written in them.
Furthermore, the news clippings didn’t have the traditional rectangular shape, but they were cut in a triangular shape, and the dagger went through them by their center.
Everything made them think that the criminal was a person who liked Mathematics, and that, she/he had left some clues whose resolution will lead them to the assassin.
Therefore, Joe Vitruvius (V) contribution seemed fundamental to solve the case, because he was an expert in the resolution of such enigmas.
The inspector got into work. He took the scraps, and read the first one: ‘For tonight’s match, our national coach hesitates which pair of central defenders will pick among the 4 available players’. On the upper part of the scrap, there was a mysterious note in red:
(4 2).
F: I don’t understand the relation between this news of the defenders and the murdered player, who is a forward. Moreover, what will these numbers in red mean, 4 and 2, written one below the other, and in brackets? –inspector Larry Flanagan said.
V: It could be a mathematical notation, a binomial coefficient, which represents the combinations of 2 elements taken from a set of 4 items. In this case, it can indicate the number of possible combinations of 2 central defenders that the coach can pick if he has 4 players available to play in this position. As we would say in our Department, combinations (without repetition) are the number of subsets of k distinct elements that we can extract from a set of n elements. – Joe Vitruvius answered.
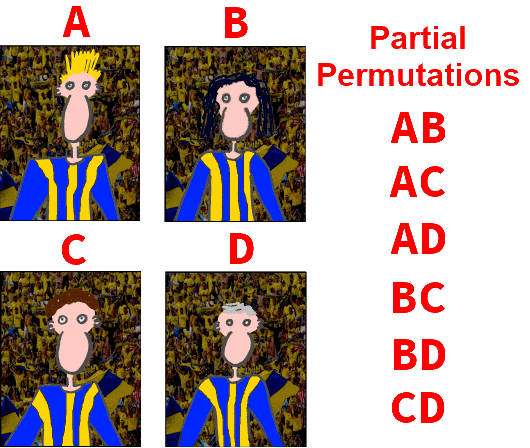
F: I see. If we have 4 players, whom we’ll call A, B, C and D (Transylvanian names are really complicated, so we’ll better use these letters), the coach may choose the following pairs of players: AB, BC, CD, AC, BD, and AD. This is, a total of 6 different pairs, right?
V: Right. This will be that way if we don’t mind what position they play. That is, it doesn’t matter if each player plays on the right or on the left of the pitch. In other case, we’d have a total of 12 combinations, because we should count the same pairs, but changing their order: BA, CB, DC, CA, DB, and DA. This way, we’d talk about partial permutations (without repetition) of 2 elements taken from a set of 4 items, and not about ‘combinations’.
F: Well, it seems that the first clue leads us to number 6. Let’s see the next scrap: ‘Indignation in Brașov. After the good season of the Sporting of Brașov, the coach will only field 2 of the 7 players of the team, that he took with him to Brazil’. Also in this clipping somebody has written a 7 and a 2, in brackets and one above the other.
F: Don’t tell me, Joe. I think this time I’ve found it by myself: the notation (7 2) represents the number of possible combinations of 2 elements taken from a set of 7 players, that the coach can pick. This is, the number of possible different pairs of players from Sporting that the coach may choose to play the match. Am I wrong?
V: No, you aren’t, inspector Larry. I see that you learn very quickly.
F: Ok, let’s see the pairs we can get. If we have 7 players, whom we’ll call A, B, C, D, E, F and G, their combinations will be: AB, FE, AC, CD, AD, BD, CF...
F: Oops! I think I’ve lost. Now it’s no so easy to calculate them... I’m going to start again, in a more ordered way: AB, AC, AD, AE....
After a while, the inspector comes to the result:
AB AC AD AE AF AG BC BD BE BF BG CD CE CF CG DE DF DG EF EG FG
F: I think we have 21 pairs, but I’m not absolutely sure that I haven’t forgotten any…
V: Yes, that’s right. Nevertheless, in this case, it would be better to use Mathematics. The formula for calculating the combinations of k elements taken from a group of n elements, is as follows:
F: Where does this formula come from?
V: Well, let’s think on the first player that the coach can choose. Clearly he has 7 possible candidates, the 7 players from Sporting. When he’s going to choose the second, he only has 6 players to pick, right? Therefore, for each of the 7 players that can be chosen initially, there will be 6 possible playmates. So we have 7 x 6 = 42 possible pairs:
AB AC AD AE AF AG BA BC BD BE BF BG CA CB CD CE CF CG DA DB DC DE DF DG EA EB EC ED EF EG FA FB FC FD FE FG
V: But in this case we get again the partial permutations of 2 elements taken from a set of 7 elements, as we have repeated pairs: AB and BA, for instance. And we said that we don’t care the position in which they play. What could we do to remove them from our formula?
F: I can’t imagine how...
V: We should divide the result by the number of possible ways we can find the same combination of players, but in a different order. It’s what, in our Department, we call ‘permutations’ (without repetition): the number of distinct ordinations we can make in a set of elements, using all the numbers, but not repeating them.
F: And how do we calculate the number of permutations?
V: It’s quite simple: If we have k elements, we have to think that any of them can be the first one. Then, there will be (k-1) elements that can be in second place (as we can’t repeat them). There will be (k-2) elements available for the third place. And so on. The total of different ways we can sort k elements will be
P(k) = k • (k-1) • (k-2) • … • 3 • 2 • 1
V: In our Department we don’t like wasting time when writing such long formulas, so we invented a secret key for this product: ‘factorial of k’, that we write: k! So we can reduce our formula:
P(k) = k!
V: For our case with sets of 2 players, we’ll have that k! = 2! = 2 • 1 = 2
V: So now we have the result for our problem. To obtain the number of combinations of 2 elements taken from a set of 7, we have to divide the number of variations of 2 elements taken from a set of 7 items by the permutations of 2 items:
F: That’s what I said at the beginning, without all these formulas!
V: It’s true, but you spent a lot of time to get the result. And there were only 7 players. What would happen if you have to choose, for example, 5 players from the 23 selected? Would you write all possible combinations?
F: Hummm… Ok. Continue your explanation, please.
V: Now, let’s modify slightly the precedent formula, by multiplying the numerator and the denominator by 5!
V: We’ve arrived to the initial formula, can you see it?
F: Correct. But I’m a little tired about all these figures, combinations, permutations, partial permutations… I think we’d better take the finger prints from the dagger, and we’ll solve the case more quickly.
V: Inspector, you know that assassins tend to wear gloves in these cases… Moreover, because of the celebration of the World Cup, the Laboratory is understaffed. And we know that they’re not so quickly in their analysis as we wanted.
F: All right. Let’s go on with our investigation. We take the third scrap: ‘Today everybody expects a hard fight in midfield, so the coach says he will choose 5 of the 7 midfield players he has brought for the World Cup.’ Above this news we can read another note (7 5).
F: Well, now we have a combination of 5 players taken from a total of 7, haven’t we? Let’s calculate it with the formula you have taught to me.
V: It’s also 21.
F: How did you calculate it so quickly?
V: I haven’t done any calculation, indeed. We have that
V: In general terms,
F: And why do we get the same result?
V: Well, you have to think that we can express in other terms the problem of calculating the number of possible combinations of 5 players if we have a total of 7 players. We can think about the 2 players that remain on the team bench each time the coach picks 5 players to enter the pitch.
ABCDE – FG
ABCDF – EG
ABCEF – DG
ABCEG – DF
…
V: That way, for each group of 5 players selected to play we have a set of 2 players not selected. There will be as many distinct groups of 5 line-up players than groups of 2 players that stay on the bench.
V: So, instead of calculating how many groups of 5 starter players we can form, we can see how many groups of 2 reserve players we can get, if we have a total of 7 players, since the solutions will be the same. And as in the previous clipping we’ve already calculated that there were 21 possible combinations for this case, now we don’t need to calculate it again.
F: Perfect. Now we only have to fit the pieces we have. The results of the 3 mysterious parentheses (4 2),(7 2) and (7 5) turned out to be numbers 6, 21 and 21. I think that if the dagger went through the 3 scraps by their centre, maybe we should find the arithmetical ‘center’ of the obtained numbers, that is, their average:
F: It seems that the murderer is a fan of Mathematics and of logic problems, and he’s going to make it difficult to us. And now, Joe, what can we do?
V: There’s a data that we haven’t used yet in our investigation, perhaps the most obvious: the scraps have a triangular shape.
V: If the murderer is a fond of Mathematics, and also if all the problems on the scraps are related to the discipline of Combinatorics, no doubt all leads us to the Pascal’s triangle. Do you know this triangle, Flanagan?
F: No, I don’t know what it is.
V: That’s normal. This triangle, as the cleverest criminals, adopts a lot of distinct personalities. For example, it’s also known as Tartaglia’s triangle. Anyway, and returning to our case, I’ll tell you that it’s a triangle very easy to draw up. First we write an 1 in the superior vertex, and then, above it, successively and in diagonal, we write the result of adding the 2 numbers above it. If there’s no number, we add a zero.

V: Now, we’re going to point, in this Pascal triangle, the results obtained. Look, inspector. In the first case (4 2), we take the fifth row (we leave out the first lonely 1) and we go to the third number, so we obtain the value 6. The same result as we get with our formula.
.jpg)
F: Why have you pointed put the third number on the fifth row, and not the second number on the fourth row?
V: Because the apex of the triangle corresponds to binomial coefficient (0 0). And, on each row n, the first number represents the combination (n 0). Thus, on our fifth line (4 0) is equal to 1, (4 1) is equal to 4, (4 2) is equal to 6, and so on. Agreed?
F: Yes. I didn’t think on number 0.
V: Now we’re going to do the same with (7 2) and (7 5). You can check that the 3 numbers make an awesome triangle, with a similar shape as the news clippings.

F: And as the triangle is symmetrical, now I understand why (7 2) is equal to (7 5).
V: Fabulous. Now, we only have to point out the number which is located at the geometrical center of the triangle we have drawn, because the dagger crossed the papers through their center. And we get number 20.

F: Which player has this number? Go and arrest him! – Inspector Larry Flanagan cried.
It was Vladimiric. The police didn’t take much time to catch him inside the stadium, and brought him to the locker room.

There, Vladimiric confessed his crime. He was Drakulic’s best friend since childhood. Drakulic had overcome him in every aspect of life: he had better academical records, was luckier with girls, had more friends and played football better. In fact, Drakulic was the captain of the national team of Transylvania, and Vladimiric was a reserve player, he played only a few minutes per match. But none of these things had been the cause that has lead to the fatal destiny.
F: And then, why have you done it? - Larry Flanagan asked him.
Well, Vladimiric said, there was only a pair of things in which Drakulic had never surpassed me: Mathematics and Logic problems. At least until today... When we were coming on the bus from the team hotel to the stadium, we made a bet on who was going to solve sooner the sudoku on the newspaper. And for the first time in our lives, Drakulic has been faster than me. I couldn’t bear it...











.gif)










No comments :
Post a Comment